| AN ARMCHAIR VIEW OF ESCALATORS
Roger W. Garrison
There is much pedagogical value in applying the economist's standard
analytical tools to issues about which students have a common-sense understanding.
By demonstrating this point repeatedly, Steven Landsburg has become the
profession's pre-eminent Armchair Economist (Landsburg, 1993). "Why
Popcorn Costs More at the Movies" (pp. 157-167) may not be a critical issue
in its own right, but dealing with such light-weight issues can be an effective
way of teaching analytical skills.
 More recently, Landsburg (Slate,
2002) has dealt with the downright frivolous question: Why don't people
walk up escalators? Or alternatively, Why don't people stand still on stairs.
Landsburg's answer, which he attributes to Mark Bils, involves taking escalators
and stairs to be instances of superior and inferior machines. Just as workers
should spend less time with an inferior machine, people should spend less
time on stairs. Well, standing still on stairs clearly violates that maxim.
More recently, Landsburg (Slate,
2002) has dealt with the downright frivolous question: Why don't people
walk up escalators? Or alternatively, Why don't people stand still on stairs.
Landsburg's answer, which he attributes to Mark Bils, involves taking escalators
and stairs to be instances of superior and inferior machines. Just as workers
should spend less time with an inferior machine, people should spend less
time on stairs. Well, standing still on stairs clearly violates that maxim.
While Bils and Landsburg get points here for
matching answer to question in terms of the degree of frivolity, they forgo
the opportunity to showcase the economic way of thinking by applying standard
indifference-curve analysis.
RESTING AND MOVING
The relevant decision, undoubtedly made subconsciously by the typical
stair climber or escalator rider, involves a trade-off between "resting"
and "moving." These two gerunds, then, label the vertical axis (resting)
and the horizontal axis (moving) of the indifference-curve map in Figure
1. The ultimate form of resting in this context consists of standing still,
which is the level of rest that defines the vertical intercept of a linear
budget constraint. (A possible budget-constraint non-linearity in the case
of moving walkways will be considered below.) The horizontal intercept
corresponds to zero rest and has our stair climber racing as fast as possible.
We should let this budget constraint be applicable only to ascending the
stairs; the constraint applicable to descending would have a horizontal
intercept lying further to the right. People can race down the stairs faster
than they can race up them. (1) In either
application and except in extreme circumstances, the actual trade-off will
be struck somewhere between standing still and racing as fast as possible.
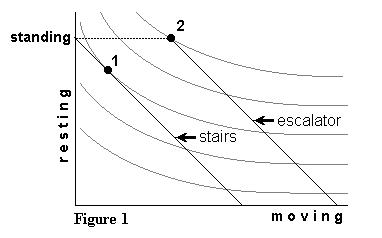 Straightforwardly, the indifference curve map shows our ascender's preferences
when faced with a choice between resting more and moving faster. A tangency
between the budget constraint and an indifference curve occurs at Point
1, which identifies the optimal combination of resting and moving on the
stairway.
Straightforwardly, the indifference curve map shows our ascender's preferences
when faced with a choice between resting more and moving faster. A tangency
between the budget constraint and an indifference curve occurs at Point
1, which identifies the optimal combination of resting and moving on the
stairway.
If the stairway is replaced by an escalator,
our budget constraint must be replaced as well. The new constraint has
the same slope as the old one but is shifted to the right by a magnitude
representing the speed of the escalator. Having the same slope simply means
that walking up an escalator and walking up stairs are equally unrestful
activities. (If walking up an escalator is judged to be more unrestful—because
of the higher steps and less suitable rise-to-run ratio—then the budget
constraint for the escalator would be a little steeper than the one for
the stairs.)
Important for application in normal circumstances
is the fact that the new budget constraint is truncated at the level of
rest associated with standing still. The constraint does not extend upward
from that point toward the vertical axis. This is only to say that you
cannot improve on the restfulness associated with standing still by walking
or running backwards on the escalator. Thus, the point that represents
standing still and moving at escalator speed is a potential corner solution.
The preference map shows that this corner, Point 2, is in fact the optimal
choice for our typical rider.
We see from Figure 1 that our climber-cum-rider
deals with the gain offered by an escalator in conventional ways. The gain
is taken partly in the form of more rest and partly in the form of more
speed. The corner solution implies that some riders would actually go further
in trading speed for rest at the margin if that were technologically possible.
But given their actual options, they are constrained to move at escalator
speed while just standing there.
 Wouldn't people be better off with escalators that allowed for tangency
solutions? The general shape of the indifference curves suggests achieving
a tangency would require escalators to move more slowly. Figure 2 shows
the case where the escalator moves at the optimal stair-climbing speed
established in Figure 1. With the potential corner solution directly above
Point 1, the typical rider would not adopt that corner as a solution. To
stand still on the slow-moving escalator would imply an indifference curve
that is perfectly vertical between Point 1 and the corner. Most everyone
would walk up—with some, presumably, ascending faster than others. Note,
however, that the tangency solution (at Point 2') entails a degree of utility
that is less than that associated with the corner solution in Figure 1.
Riders are better off with a corner solution on a faster-moving escalator.
Wouldn't people be better off with escalators that allowed for tangency
solutions? The general shape of the indifference curves suggests achieving
a tangency would require escalators to move more slowly. Figure 2 shows
the case where the escalator moves at the optimal stair-climbing speed
established in Figure 1. With the potential corner solution directly above
Point 1, the typical rider would not adopt that corner as a solution. To
stand still on the slow-moving escalator would imply an indifference curve
that is perfectly vertical between Point 1 and the corner. Most everyone
would walk up—with some, presumably, ascending faster than others. Note,
however, that the tangency solution (at Point 2') entails a degree of utility
that is less than that associated with the corner solution in Figure 1.
Riders are better off with a corner solution on a faster-moving escalator.
Providing answers to the original inquiry
(Why don't people walk up escalators?) leads us to a related question—a
question that has a more satisfying answer: On what basis do escalator
manufactures set the speed of their escalators? It would seem that they
set the speed at a level that puts most riders at a corner solution.
(2) With most riders standing still, the conflicts among riders
are nullified. Further, the dominance of the corner solution justifies
an escalator design that best accommodates standers. (The slow-moving escalator
of Figure 2 should have a step height and rise-to-run ratio of a conventional
stairway. That design would best accommodate the climbers.)
Even with the faster-moving escalator of Figure
1, some people will climb. Their preferences imply a tangency solution.
Some may even climb as rapidly as they would climb stairs. These people
are simply taking all of the gain provided by the escalator in the form
of speed and none of the gain in the form of rest.
(3) It is probably the case that an escalator speed set so high
that literally no one would walk or run up it is set so high that virtually
no one would get on it. Further, we see in the following section that it
is no contradiction of economic theory for some people in some circumstances
to move faster up an escalator than they would move on stairs.
TRAINS, PLANES, AND CONVENTION HOTELS
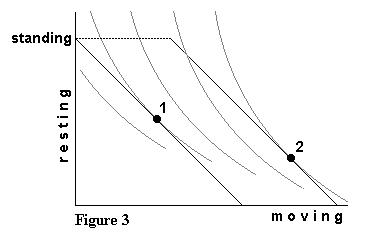
Figure 3 is identical to Figure 1 in terms of the shape and location
of the budget constraints, but it differs from the earlier figure in terms
of the preference map. Given the particular indifference curves of Figure
3, we get a tangency solution in which the rider actually leverages the
gain provided by the escalator. As implied by a movement from Point 1 to
Point 2, he runs up it, though with stairs he would only have walked up.
We can easily imagine the circumstances in which these preferences are
understandable. Suppose it is very much worth while to get to the next
floor quickly but that if you can't get there quickly, it doesn't much
matter whether you get there a little later or even later still. Train
stations and airports provide circumstances where these indifference curves
might apply.
 The escalator may give the traveler just the leverage he or she needs to
catch an on-time plane. But absent the escalator, all hope is lost. The
stair-climber walks up the stairs at a normal pace—on the chance that the
train/plane is late or to check the time table for the next departure.
(Undoubtedly, some of these travelers might have run up the stairs. Still,
the case in which a traveler would walk up the stairs but run up the escalator
is conceivable and even plausible.) The behavior motivated by the preference
map in Figure 3 is probably common in train stations and airports but rare
to non-existent in convention hotels. Convention participants are not terribly
concerned about missing the first few minutes of some session on the application
of indifference-curve analysis.
The escalator may give the traveler just the leverage he or she needs to
catch an on-time plane. But absent the escalator, all hope is lost. The
stair-climber walks up the stairs at a normal pace—on the chance that the
train/plane is late or to check the time table for the next departure.
(Undoubtedly, some of these travelers might have run up the stairs. Still,
the case in which a traveler would walk up the stairs but run up the escalator
is conceivable and even plausible.) The behavior motivated by the preference
map in Figure 3 is probably common in train stations and airports but rare
to non-existent in convention hotels. Convention participants are not terribly
concerned about missing the first few minutes of some session on the application
of indifference-curve analysis.
Shopping malls are usually like convention
hotels but are sometimes like train stations and airports. On the day after
Christmas or on other special sales days, it is critical to get to the
merchandise ahead of the crowd; but failing that, it will do just to see
what's left over after the mad scramble. Shoppers whose preferences are
more conventional—i.e., similar to the ones shown in Figure 1—might want
to be put on notice that there are other shoppers among them whose preference
maps are similar to the one shown in Figure 3. Possibly it would be worthwhile
to create an iconic symbol that resembles the pattern of indifference curves
of Figure 3 and post it near the mall entrance on sales days. The notice
could serve a function similar to a posting at the beach that warns of
a rip tide.
MOVING WALKWAYS
 An indifference-curve
treatment of moving walkways would seem to be similar in all respects to
our treatment of escalators. But there are critical differences. The budget
constraint has a much shallower slope and possibly is non-linear near the
vertical intercept, virtually precluding the kind of dominant corner solution
that characterizes our analysis of escalators. Figure 4 shows indifference
curves from the same preference map used in Figure 1. But the budget constraint
has a slope that is considerably less than in Figure 1: On a level playing
field, people can trade rest for speed on much more favorable terms. An indifference-curve
treatment of moving walkways would seem to be similar in all respects to
our treatment of escalators. But there are critical differences. The budget
constraint has a much shallower slope and possibly is non-linear near the
vertical intercept, virtually precluding the kind of dominant corner solution
that characterizes our analysis of escalators. Figure 4 shows indifference
curves from the same preference map used in Figure 1. But the budget constraint
has a slope that is considerably less than in Figure 1: On a level playing
field, people can trade rest for speed on much more favorable terms.
The shallow slope alone makes it unlikely
that a corner solution will dominate. In Figure 4, our typical rider takes
advantage of a moving walkway by locating at Point 2, which constitutes
a tangency solution. It is without contradiction, then, that many people
(including the author) stand on an escalator  but
walk on a moving walkway. Making a corner solution even less likely is
the fact that for many, strolling may seem more restful than standing.
(Strolling around a shopping mall certainly seems less tiring than just
standing in one of the stores.) If strolling is preferred to walking even
on grounds of restfulness, then the budget constraint itself rises
from its vertical intercept and then slopes downward at speeds beyond the
stroll. As is clear in Figure 5, this kind of non-linearity would preclude
a corner solution. but
walk on a moving walkway. Making a corner solution even less likely is
the fact that for many, strolling may seem more restful than standing.
(Strolling around a shopping mall certainly seems less tiring than just
standing in one of the stores.) If strolling is preferred to walking even
on grounds of restfulness, then the budget constraint itself rises
from its vertical intercept and then slopes downward at speeds beyond the
stroll. As is clear in Figure 5, this kind of non-linearity would preclude
a corner solution.
Of course, some people do stand on moving
walkways. Standing may even be typical for riders who have luggage or are
otherwise encumbered. Others stroll for added restfulness or to avoid boredom.
Still others walk, taking only part of the gain provided by the moving
walkway in the form of rest, or they walk fast, leveraging the gain. Without
a corner solution to fix a dominant mode of usage, measures need to be
taken to deal with the variety of modes. Typically, riders are reminded
by conspicuous signs or by a taped voice to stand on the right or walk
on the left. Though less common (except possibly in England), this same
convention can be prescribed for escalator riders.
SUMMARY
Using indifference curve analysis to show why people stand still on
escalators but walk on moving walkways helps establish the near-universal
applicability of economic theory. Working with contrasting preference maps
(such as those in Figures 1 and 3) to deal with an issue where the student's
own intuition is fully in play may help the student to read indifference
curves in less intuitive cases. And challenging the students to apply basic
economic tools to similarly frivolous issues can result in fun and even
learning.
The only down side to exposing students to
this armchair view of escalators is that they may never again be able to
ride an airport escalator without thinking of indifference-curve analysis.
REFERENCES
Landsburg, S. E. (2002). Everyday Economics: "Why do you
walk up staircases but not up escalators? Slate (http://slate.msm.com),
August 28.
Landsburg, S. E. (1993). The Armchair Economist: Economics
and Everyday Life. New York: The Free Press.
NOTES
1. One of my colleagues whose armchair
is much newer than my own claims that she can go up the stairs (taking
three steps at a time) faster than she can go down (having to use every
step).
2. Actually polling the manufactures
of escalators on this question, of course, would violate the spirit of
armchair theorizing. In any case, we can claim they behave as if
they have a corner solution in mind.
3. According to Landsburg (2002), the
Bils-Landsburg argument "proves...that even if you choose to walk on the
escalator, you should always walk even faster on the stairs" (emphasis
added). The "always," it turns out, makes their statement too strong.
|
 More recently, Landsburg (
More recently, Landsburg ( Straightforwardly, the indifference curve map shows our ascender's preferences
when faced with a choice between resting more and moving faster. A tangency
between the budget constraint and an indifference curve occurs at Point
1, which identifies the optimal combination of resting and moving on the
stairway.
Straightforwardly, the indifference curve map shows our ascender's preferences
when faced with a choice between resting more and moving faster. A tangency
between the budget constraint and an indifference curve occurs at Point
1, which identifies the optimal combination of resting and moving on the
stairway.
 Wouldn't people be better off with escalators that allowed for tangency
solutions? The general shape of the indifference curves suggests achieving
a tangency would require escalators to move more slowly. Figure 2 shows
the case where the escalator moves at the optimal stair-climbing speed
established in Figure 1. With the potential corner solution directly above
Point 1, the typical rider would not adopt that corner as a solution. To
stand still on the slow-moving escalator would imply an indifference curve
that is perfectly vertical between Point 1 and the corner. Most everyone
would walk up—with some, presumably, ascending faster than others. Note,
however, that the tangency solution (at Point 2') entails a degree of utility
that is less than that associated with the corner solution in Figure 1.
Riders are better off with a corner solution on a faster-moving escalator.
Wouldn't people be better off with escalators that allowed for tangency
solutions? The general shape of the indifference curves suggests achieving
a tangency would require escalators to move more slowly. Figure 2 shows
the case where the escalator moves at the optimal stair-climbing speed
established in Figure 1. With the potential corner solution directly above
Point 1, the typical rider would not adopt that corner as a solution. To
stand still on the slow-moving escalator would imply an indifference curve
that is perfectly vertical between Point 1 and the corner. Most everyone
would walk up—with some, presumably, ascending faster than others. Note,
however, that the tangency solution (at Point 2') entails a degree of utility
that is less than that associated with the corner solution in Figure 1.
Riders are better off with a corner solution on a faster-moving escalator.
 The escalator may give the traveler just the leverage he or she needs to
catch an on-time plane. But absent the escalator, all hope is lost. The
stair-climber walks up the stairs at a normal pace—on the chance that the
train/plane is late or to check the time table for the next departure.
(Undoubtedly, some of these travelers might have run up the stairs. Still,
the case in which a traveler would walk up the stairs but run up the escalator
is conceivable and even plausible.) The behavior motivated by the preference
map in Figure 3 is probably common in train stations and airports but rare
to non-existent in convention hotels. Convention participants are not terribly
concerned about missing the first few minutes of some session on the application
of indifference-curve analysis.
The escalator may give the traveler just the leverage he or she needs to
catch an on-time plane. But absent the escalator, all hope is lost. The
stair-climber walks up the stairs at a normal pace—on the chance that the
train/plane is late or to check the time table for the next departure.
(Undoubtedly, some of these travelers might have run up the stairs. Still,
the case in which a traveler would walk up the stairs but run up the escalator
is conceivable and even plausible.) The behavior motivated by the preference
map in Figure 3 is probably common in train stations and airports but rare
to non-existent in convention hotels. Convention participants are not terribly
concerned about missing the first few minutes of some session on the application
of indifference-curve analysis.
 An indifference-curve
treatment of moving walkways would seem to be similar in all respects to
our treatment of escalators. But there are critical differences. The budget
constraint has a much shallower slope and possibly is non-linear near the
vertical intercept, virtually precluding the kind of dominant corner solution
that characterizes our analysis of escalators. Figure 4 shows indifference
curves from the same preference map used in Figure 1. But the budget constraint
has a slope that is considerably less than in Figure 1: On a level playing
field, people can trade rest for speed on much more favorable terms.
An indifference-curve
treatment of moving walkways would seem to be similar in all respects to
our treatment of escalators. But there are critical differences. The budget
constraint has a much shallower slope and possibly is non-linear near the
vertical intercept, virtually precluding the kind of dominant corner solution
that characterizes our analysis of escalators. Figure 4 shows indifference
curves from the same preference map used in Figure 1. But the budget constraint
has a slope that is considerably less than in Figure 1: On a level playing
field, people can trade rest for speed on much more favorable terms.
 but
walk on a moving walkway. Making a corner solution even less likely is
the fact that for many, strolling may seem more restful than standing.
(Strolling around a shopping mall certainly seems less tiring than just
standing in one of the stores.) If strolling is preferred to walking even
on grounds of restfulness, then the budget constraint itself rises
from its vertical intercept and then slopes downward at speeds beyond the
stroll. As is clear in Figure 5, this kind of non-linearity would preclude
a corner solution.
but
walk on a moving walkway. Making a corner solution even less likely is
the fact that for many, strolling may seem more restful than standing.
(Strolling around a shopping mall certainly seems less tiring than just
standing in one of the stores.) If strolling is preferred to walking even
on grounds of restfulness, then the budget constraint itself rises
from its vertical intercept and then slopes downward at speeds beyond the
stroll. As is clear in Figure 5, this kind of non-linearity would preclude
a corner solution.